Symmetric relation

A symmetric relation is a type of binary relation. An example is the relation "is equal to", because if a = b is true then b = a is also true. Formally, a binary relation R over a set X is symmetric if:

\( {\displaystyle \forall a,b\in X(aRb\Leftrightarrow bRa).} \)

If RT represents the converse of R, then R is symmetric if and only if R = RT.

Symmetry, along with reflexivity and transitivity, are the three defining properties of an equivalence relation.

Examples

In mathematics

"is equal to" (equality) (whereas "is less than" is not symmetric)
"is comparable to", for elements of a partially ordered set
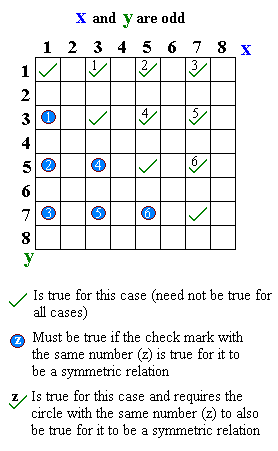
"... and ... are odd":

Outside mathematics

"is married to" (in most legal systems)
"is a fully biological sibling of"
"is a homophone of"
"is co-worker of"
"is teammate of"

Relationship to asymmetric and antisymmetric relations

By definition, a nonempty relation cannot be both symmetric and asymmetric (where if a is related to b, then b cannot be related to a (in the same way)). However, a relation can be neither symmetric nor asymmetric, which is the case for "is less than or equal to" and "preys on").

Symmetric and antisymmetric (where the only way a can be related to b and b be related to a is if a = b) are actually independent of each other, as these examples show.

Mathematical examples
	Symmetric	Not symmetric
Antisymmetric	equality	"is less than or equal to"
Not antisymmetric	congruence in modular arithmetic	"is divisible by", over the set of integers

Non-mathematical examples
	Symmetric	Not symmetric
Antisymmetric	"is the same person as, and is married"	"is the plural of"
Not antisymmetric	"is a full biological sibling of"	"preys on"

Properties

A symmetric and transitive relation is always quasireflexive.

A symmetric, transitive, and reflexive relation is called an equivalence relation.

One way to conceptualize a symmetric relation in graph theory is that a symmetric relation is an edge, with the edge's two vertices being the two entities so related. Thus, symmetric relations and undirected graphs are combinatorially equivalent objects.