In mathematics, the Pearcey[1] integral[2] is defined as[3]
\operatorname {Pe}(x,y)=\int _{{-\infty }}^{\infty }\exp(i(t^{4}+xt^{2}+yt))\,dt.
The Pearcey integral is a class of canonical diffraction integrals, often used in wave propagation and optical diffraction problems[4]
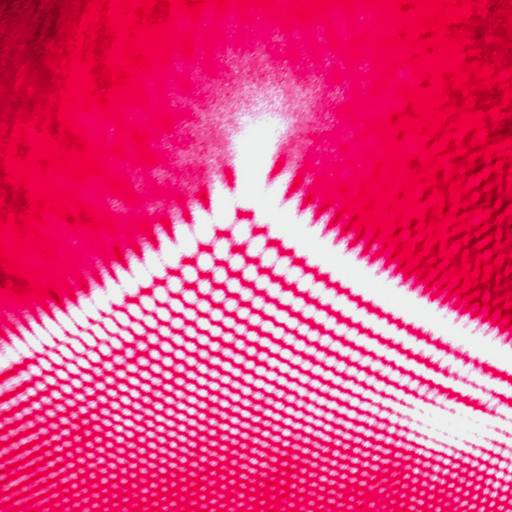
In optics, the Pearcey integral can be used to model diffraction effects at a cusp caustic.
A photograph of a cusp caustic produced by illuminating a flat surface with a laser beam through a droplet of water.
Graphs
A plot of the absolute value of the Pearcey integral as a function of its two parameters.
References
https://csiropedia.csiro.au/Pearcey-Trevor/
T. Pearcey, The structure of an electromagnetic field in the neighbourhood of a cusp of a caustic, The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 37, 311-317, 1946
Frank W. J. Olver, Daniel W. Lozier, Ronald F. Boisvert, Charles W. Clark, NIST Handbook of Mathematical Functions, p. 777, Cambridge, 2010
R.B.Paris, Hadamard Expansions and Hyperasymptotic Evaluation, p. 207, Encyclopedia of Mathematics and its Applications, 141, Cambridge, 2011
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Hellenica World - Scientific Library
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License