In geometry, the order-5 square tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {4,5}.
Related polyhedra and tiling
Spherical Hyperbolic tilings
| Spherical | Hyperbolic tilings
|
|||||||
|---|---|---|---|---|---|---|---|---|
 {2,5} |
 {3,5} |
 {4,5} |
 {5,5} |
 {6,5} |
 {7,5} |
 {8,5} |
... |  {∞,5} |
This tiling is topologically related as a part of sequence of regular polyhedra and tilings with vertex figure (4n).
*n42 symmetry mutation of regular tilings: {4,n}
| Uniform pentagonal/square tilings | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [5,4], (*542) | [5,4]+, (542) | [5+,4], (5*2) | [5,4,1+], (*552) | ||||||||
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
||
| {5,4} | t{5,4} | r{5,4} | 2t{5,4}=t{4,5} | 2r{5,4}={4,5} | rr{5,4} | tr{5,4} | sr{5,4} | s{5,4} | h{4,5} | ||
| Uniform duals | |||||||||||
 |
 |
 |
 |
 |
 |
 |
 |
 |
|||
| V54 | V4.10.10 | V4.5.4.5 | V5.8.8 | V45 | V4.4.5.4 | V4.8.10 | V3.3.4.3.5 | V3.3.5.3.5 | V55 | ||
| Order-5 square tiling | |
|---|---|
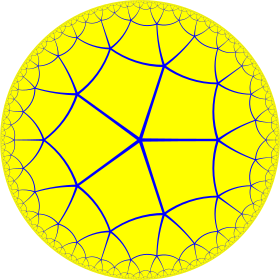 Poincaré disk model of the hyperbolic plane |
|
| Type | Hyperbolic regular tiling |
| Vertex configuration | 45 |
| Schläfli symbol | {4,5} |
| Wythoff symbol | 5 | 4 2 |
| Coxeter diagram | |
| Symmetry group | [5,4], (*542) |
| Dual | Order-4 pentagonal tiling |
| Properties | Vertex-transitive, edge-transitive, face-transitive |
This hyperbolic tiling is related to a semiregular infinite skew polyhedron with the same vertex figure in Euclidean 3-space.
References
John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
"Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
See also
Wikimedia Commons has media related to Order-5 square tiling.
Square tiling
Uniform tilings in hyperbolic plane
List of regular polytopes
Medial rhombic triacontahedron
External links
Weisstein, Eric W. "Hyperbolic tiling". MathWorld.
Weisstein, Eric W. "Poincaré hyperbolic disk". MathWorld.
Hyperbolic and Spherical Tiling Gallery
KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
Hyperbolic Planar Tessellations, Don Hatch
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Hellenica World - Scientific Library
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License


