The right triangle altitude theorem or geometric mean theorem is a result in elementary geometry that describes a relation between the lengths of the altitude on the hypotenuse in a right triangle and the two line segments it creates on the hypotenuse. It states that the geometric mean of the two segments equals the altitude.
Theorem and applications
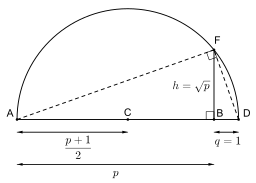
Construction of √p by setting q to 1
If h denotes the altitude in a right triangle and p and q the segments on the hypotenuse then the theorem can be stated as:[1]
\( h={\sqrt {pq}} \)
or in term of areas:
\( h^{2}=pq. \)
AM-GM inequality
The latter version yields a method to square a rectangle with ruler and compass, that is to construct a square of equal area to a given rectangle. For such a rectangle with sides p and q we denote its top left vertex with D. Now we extend the segment q to its left by p (using arc AE centered on D) and draw a half circle with endpoints A and B with the new segment p+q as its diameter. Then we erect a perpendicular line to the diameter in D that intersects the half circle in C. Due to Thales' theorem C and the diameter form a right triangle with the line segment DC as its altitude, hence DC is the side of a square with the area of the rectangle. The method also allows for the construction of square roots (see constructible number), since starting with a rectangle that has a width of 1 the constructed square will have a side length that equals the square root of the rectangle's length.[1]
The theorem can be used to provide a geometrical proof of the AM–GM inequality in the case of two numbers. For the numbers p and q one constructs a half circle with diameter p+q. Now the altitude represents the geometric mean and the radius the arithmetic mean of the two numbers. Since the altitude is always smaller or equal to the radius, this yields the inequality.[2]
geometric mean theorem as a special case of the chord theorem:
\( {\displaystyle |CD||DE|=|AD||DB|\Leftrightarrow h^{2}=pq} \)
The geometric mean theorem can also be thought of as a special case of the intersecting chords theorem for a circle, since the converse of Thales' theorem ensures that the hypotenuse of the right angled triangle is the diameter of its circumcircle.[1]
The converse statement is true as well. Any triangle, in which the altitude equals the geometric mean of the two line segments created by it, is a right triangle.
History
The theorem is usually attributed to Euclid (ca. 360–280 BC), who stated it as a corollary to proposition 8 in book VI of his Elements. In proposition 14 of book II Euclid gives a method for squaring a rectangle, which essentially matches the method given here. Euclid however provides a different slightly more complicated proof for the correctness of the construction rather than relying on the geometric mean theorem.[1][3]
Proof
Based on similarity
\( {\displaystyle \triangle ABC\sim \triangle ADC\sim \triangle DBC} \)
Proof of theorem:
The triangles \( \triangle ADC \) and \( {\displaystyle \triangle BCD} \) are similar, since:
consider triangles \( {\displaystyle \triangle ABC,\triangle ACD} \), here we have \( {\displaystyle \angle ACB=\angle ADC=90^{\circ }} \) and \( {\displaystyle \angle BAC=\angle CAD} \), therefore by the AA postulate \( {\displaystyle \triangle ABC\sim \triangle ACD} \)
further, consider triangles \( {\displaystyle \triangle ABC,\triangle BCD} \), here we have \( {\displaystyle \angle ACB=\angle BDC=90^{\circ }} \) and \( {\displaystyle \angle ABC=\angle CBD}, therefore by the AA postulate \( {\displaystyle \triangle ABC\sim \triangle BCD} \)
Therefore, both triangles \( {\displaystyle \triangle ACD} \) and \( {\displaystyle \triangle BCD} \) are similar to \( \triangle ABC \) and themselves, i.e. \( {\displaystyle \triangle ACD\sim \triangle ABC\sim \triangle BCD}. \)
Because of the similarity we get the following equality of ratios and its algebraic rearrangement yields the theorem:.[1]
\( {\frac {h}{p}}={\frac {q}{h}}\,\Leftrightarrow \,h^{2}=pq\,\Leftrightarrow \,h={\sqrt {pq}}\qquad (h,p,q>0) \)
Proof of converse:
For the converse we have a triangle \( \triangle ABC \) in which \( h^{2}=pq \) holds and need to show that the angle at C is a right angle. Now because of \( h^{2}=pq \) we also have \( {\tfrac {h}{p}}={\tfrac {q}{h}} \). Together with \( \angle ADC=\angle CDB \) the triangles \( \triangle ADC \) and \( \triangle BDC \) have an angle of equal size and have corresponding pairs of legs with the same ratio. This means the triangles are similar, which yields:
\( {\displaystyle \angle ACB=\angle ACD+\angle DCB=\angle ACD+(90^{\circ }-\angle DBC)=\angle ACD+(90^{\circ }-\angle ACD)=90^{\circ }} \)
Based on the Pythagorean theorem
Proof with the Pythagorean theorem
In the setting of the geometric mean theorem there are three right triangles \( \triangle ABC \), \( \triangle ADC \) and \( {\displaystyle \triangle DBC} \), in which the Pythagorean theorem yields:
\( {\displaystyle h^{2}=a^{2}-q^{2}} \), \( {\displaystyle h^{2}=b^{2}-p^{2}} \) and \( c^{2}=a^{2}+b^{2} \)
Adding the first 2 two equations and then using the third then leads to:
\( {\displaystyle 2h^{2}=a^{2}+b^{2}-p^{2}-q^{2}=c^{2}-p^{2}-q^{2}=(p+q)^{2}-p^{2}-q^{2}=2pq}. \)
A division by two finally yields the formula of the geometric mean theorem.[4]
Based on dissection and rearrangement
Dissecting the right triangle along its altitude h yields two similar triangles, which can be augmented and arranged in two alternative ways into a larger right triangle with perpendicular sides of lengths p+h and q+h. One such arrangement requires a square of area h2 to complete it, the other a rectangle of area pq. Since both arrangements yield the same triangle, the areas of the square and the rectangle must be identical.
Based on shear mappings
The square of the altitude can be transformed into an rectangle of equal area with sides p and q with the help of three shear mappings (shear mappings preserve the area):
Shear mappings with their associated fixed lines (dotted), starting with the original square as preimage each parallelogram displays the image of a shear mapping of the figure left of it
References
*Hartmut Wellstein, Peter Kirsche: Elementargeometrie. Springer, 2009, ISBN 9783834808561, pp. 76-77 (German, online copy, p. 76, at Google Books)
Claudi Alsina, Roger B. Nelsen: Icons of Mathematics: An Exploration of Twenty Key Images. MAA 2011, ISBN 9780883853528, pp. 31–32 (online copy, p. 31, at Google Books)
Euclid: Elements, book II – prop. 14, book VI – prop. 8, (online copy)
Ilka Agricola, Thomas Friedrich: Elementary Geometry. AMS 2008, ISBN 9780821843475, p. 25 (online copy, p. 25, at Google Books)
External links
Geometric Mean at Cut-the-Knot
Ancient Greek and Hellenistic mathematics (Euclidean geometry)
Mathematicians
(timeline)
Anaxagoras Anthemius Archytas Aristaeus the Elder Aristarchus Apollonius Archimedes Autolycus Bion Bryson Callippus Carpus Chrysippus Cleomedes Conon Ctesibius Democritus Dicaearchus Diocles Diophantus Dinostratus Dionysodorus Domninus Eratosthenes Eudemus Euclid Eudoxus Eutocius Geminus Heliodorus Heron Hipparchus Hippasus Hippias Hippocrates Hypatia Hypsicles Isidore of Miletus Leon Marinus Menaechmus Menelaus Metrodorus Nicomachus Nicomedes Nicoteles Oenopides Pappus Perseus Philolaus Philon Philonides Porphyry Posidonius Proclus Ptolemy Pythagoras Serenus Simplicius Sosigenes Sporus Thales Theaetetus Theano Theodorus Theodosius Theon of Alexandria Theon of Smyrna Thymaridas Xenocrates Zeno of Elea Zeno of Sidon Zenodorus
Treatises
Almagest Archimedes Palimpsest Arithmetica Conics (Apollonius) Catoptrics Data (Euclid) Elements (Euclid) Measurement of a Circle On Conoids and Spheroids On the Sizes and Distances (Aristarchus) On Sizes and Distances (Hipparchus) On the Moving Sphere (Autolycus) Euclid's Optics On Spirals On the Sphere and Cylinder Ostomachion Planisphaerium Sphaerics The Quadrature of the Parabola The Sand Reckoner
Problems
Angle trisection Doubling the cube Squaring the circle Problem of Apollonius
Concepts/Definitions
Circles of Apollonius
Apollonian circles Apollonian gasket Circumscribed circle Commensurability Diophantine equation Doctrine of proportionality Golden ratio Greek numerals Incircle and excircles of a triangle Method of exhaustion Parallel postulate Platonic solid Lune of Hippocrates Quadratrix of Hippias Regular polygon Straightedge and compass construction Triangle center
Results
In Elements
Angle bisector theorem Exterior angle theorem Euclidean algorithm Euclid's theorem Geometric mean theorem Greek geometric algebra Hinge theorem Inscribed angle theorem Intercept theorem Pons asinorum Pythagorean theorem Thales's theorem Theorem of the gnomon
Apollonius
Apollonius's theorem
Other
Aristarchus's inequality Crossbar theorem Heron's formula Irrational numbers Menelaus's theorem Pappus's area theorem Ptolemy's inequality Ptolemy's table of chords Ptolemy's theorem Spiral of Theodorus
Centers
Cyrene Library of Alexandria Platonic Academy
Other
Ancient Greek astronomy Greek numerals Latin translations of the 12th century Neusis construction
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Hellenica World - Scientific Library
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License